Role of Interfilament Spacing in Muscle Filament Calcium Sensitivity

The heart regulates ventricular output in response to changes in ventricular filling, a mechanism known as Frank Starling’s law of the heart. Part of the cellular basis for the law is an increase in myofilament Ca²⁺ responsiveness upon an increase in sarcomere length (SL). (Sarcomeres are the basic functional units of muscle fibers, which contract in response to calcium influx.) How information on SL is transmitted to myofilaments is unclear. One prominent hypothesis is that a decrease in myofilament lattice spacing (LS) in response to an increase in SL underlies the Ca²⁺ sensitivity.

In support of this hypothesis, shrinkage of the myofilament lattice by osmotic compression using high-molecular-weight moieties has been shown to increase myofilament Ca²⁺ sensitivity at a short SL, mimicking the effect of increased SL. The extent of LS shrinkage in that experiment was estimated from the reduction in muscle width, however. Recently, researchers directly measured LS by synchrotron x-ray diffraction as a function of SL in skinned rat cardiac trabeculae bathed in 0-6% dextran (high-molecular-weight) solutions.

To assess the effect of SL on myofilament Ca²⁺ sensitivity, Ca²⁺-contraction force relationships were determined at three SLs (1.95, 2.10, and 2.25 μm) in each group studied (0%, 3%, and 6% dextran solution). Increasing SL caused a leftward shift of the force relationship. Although application of dextran caused a general increase in myofilament Ca²⁺ sensitivity at each SL, the length-dependent shift of the force relationship did not seem altered by dextran treatment.

Researchers simultaneously measured myofilament LS and SL by using synchrotron x-ray diffraction at the Biophysics Collaborative Access Team beamline 18-ID. Increasing dextran concentration modified the diffraction pattern of individual samples in a way indicative of a reduction in LS. However, LS became a less sensitive function of SL with increasing amounts of dextran in solution. These results indicate that the impact of interfilament spacing on myofilament Ca²⁺ sensitivity is not constant but rather varies with the overall compression state of the sarcomere.

Under the current conditions, application of 3% dextran compressed the myofilament lattice beyond that observed at even the largest SL under control conditions. Therefore, skinned trabeculae were treated with 1% dextran to osmotically compress the myofilament lattice at a short SL to match that of the uncompressed control sample at a longer SL. Application of 1% dextran to a 2.02-μm-SL sample did not significantly alter Ca²⁺ sensitivity, despite the reduction in myofilament LS.

Fig. 1 Part A shows the average myofilament LS as a function of SL in skinned cardiac trabeculae bathed in either a 0% (top, rectangles), 3% (middle, squares), or 6% (bottom, diamonds) dextran solution (n = 8 to 10). The relationships were obtained by averaging the data into 0.05-μm-wide SL bins. Part B shows the average impact of SL on myofilament LS (ΔLS) and Ca²⁺ sensitivity (ΔEC₅₀) at each dextran concentration. Note the significant differences from control group (0% dextran), P is <0.01; P is <0.01 from 3% dextran group. For this test case, the average Ca²⁺ sensitivities (as indexed by the EC₅₀ parameter), are given in C.

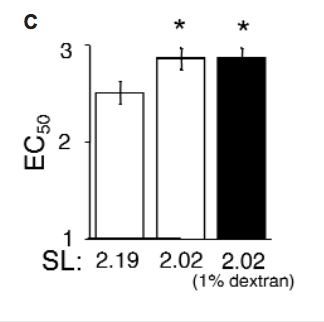
If interfilament spacing were the molecular length sensor, one would expect the reduction in length dependence of myofilament LS to lead to a reduction of the length dependence of myofilament activation. Osmotic compression in this study, however, did not affect the length dependence of myofilament Ca²⁺ sensitivity. The precise reason for the discrepancies between previous studies and the current study is unclear.

Our data suggest that, at a minimum, the interfilament spacing theory must be amended to include a variable impact of myofilament LS on Ca²⁺ sensitivity, depending on the overall extent of LS compression. At 6% dextran, LS reduction relative to that at 3% dextran was not matched by a further increase in Ca²⁺ sensitivity, in contradiction to the theory. The relationship between interfilament spacing and Ca²⁺ sensitivity may therefore be highly nonlinear. However, the 1% dextran test indicates that changes in LS per se are not responsible for increased Ca²⁺ sensitivity. If they were, the moderately compressed trabeculae at short SL should have behaved similarly to uncompressed trabeculae at long SL, given the equivalent LS.

See: J.P. Konhilas, T.C. Irving, and P. deTombe, “Myofilament calcium sensitivity in skinned rat cardiac trabeculae — Role of interfilament spacing,” Circ. Res. 90, 59-65 (2002).